回折限界

diffraction limit

[目次：レンズ系]

光学系に収差が無い場合の集光限界。電子波には回折現象があるので、収差がない光学系においても、物体の一点から出射した電子波は像面で無限小の一点には集まらず、有限の大きさ(エアリーディスク)までにしか集光できない。回折収差によるエアリーディスクの半径rはr=0.6λ/sinαである。ここで、λは電子線の波長、αはレンズの開き角である。この式から、エアリーディスクの大きさは電子線の開き角を大きくとると小さくなることがわかる。この集光限界のために理想レンズでも点分解能は無限に小さくはならない。

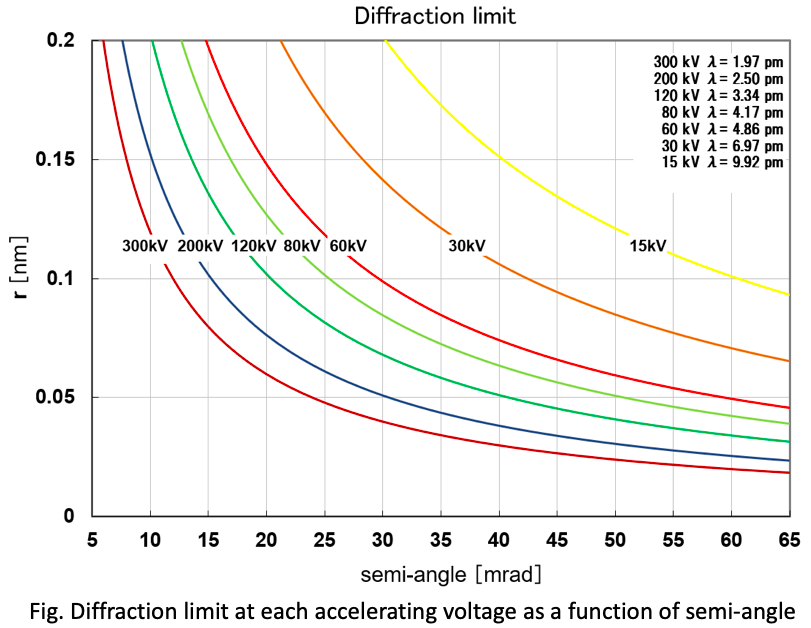
図は、開き角α(横軸)に対する各加速電圧ごとに回折限界(縦軸) r ＝ 0.6λ/sinαをグラフ化したものである。同じ開き角αでも、加速電圧が低いほど電子線の波長が長いので、回折限界rの値が大きくなる。加速電圧200kVの場合、（回折限界から見積もった）分解能0.1nmを達成するために必要な開き角は約15mradであるが、80kVの場合は、波長がさらに長くなるため約25mradの開き角が必要になる。

The diffraction limit is the resolution limit due to diffraction of an electron wave for the optical system with no aberrations. Even in the aberration-free optical system, electron waves exiting from one point on the object do not form an infinitesimal point on the image plane but these electron waves are focused into a finite size spot (airy disk) due to their diffraction phenomenon. The radius r of the airy disk is given by the equation r = 0.6λ/sinα, where λ is the wavelength of the electron and α is the divergence angle of the electron. From the equation, it is seen that the size of the airy disk is small for a large divergence angle of the electron beam. This limitation makes it impossible to produce an infinitesimal point resolution even for ideal lenses.

Figure shows the diffraction limit (vertical axis) r ＝ 0.6λ/sinα for each accelerating voltage as a function of divergence semi-angle α. Since the wavelength of an electron beam is longer for the lower accelerating voltage, the diffraction limit r becomes larger for the lower accelerating voltage even for the same semi-angle α. In the case of an accelerating voltage of 200 kV, a resolution of 0.1 nm expected from the diffraction limit is achieved with a divergence semi-angle of approximately 15 mrad. In the case of 80 kV, however, a larger divergence semi-angle of an approximately 25 mrad is required because of a longer wavelength of the electron beam.