動力学的回折

dynamical diffraction

結晶性の試料に入射した電子はブラッグ条件を満たす格子面で反射(回折)する。試料が厚いと多くの電子が反射され入射波の強度は減少し、反射波の強度が入射波の強度を上回る。ある厚さ（消衰距離の1/2）に達すると、ついには入射波の強度はゼロになり、反射波の強度が最大になる。すると今度は反射波が入射波のほうに反射され、消衰距離に達すると、再び入射波の強度が最大になり反波の強度はゼロになる。このように回折波と入射波の間の相互作用を考慮する回折現象のことを動力学的回折という。

試料が薄い場合(＜3 nm)は、反射が僅かしか起こらないと仮定して回折現象を扱うことができる。このような近似を運動学的回折という。X線回折や中性子回折では、散乱能が小さいので、運動学的回折が適用できる。

図に、反射が僅かしか起こらない運動学的な回折の様子(図a)と、多くの電子が反射を起こし入射波と反射波の間に相互作用が起きる動力学的な回折の様子を模式的に示した。

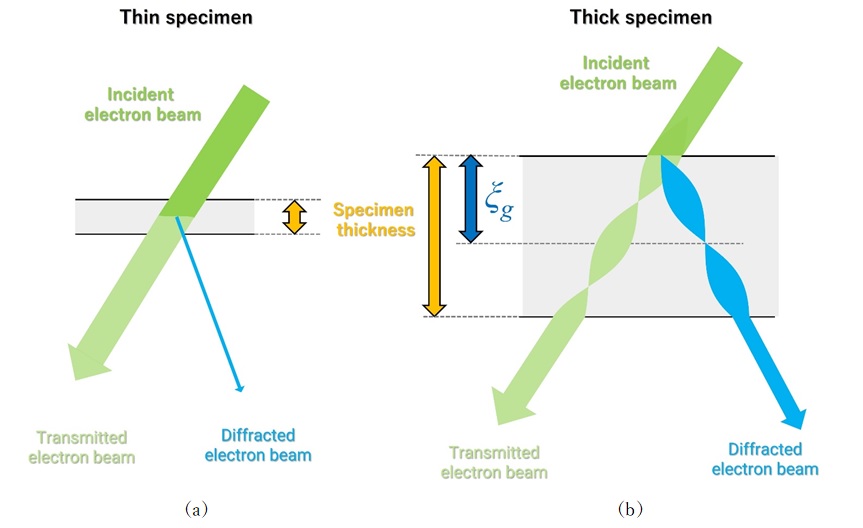
図(a）運動学的回折では、入射波からの回折は僅かしか起こらず、回折波の大きさは透過波の大きさに比べ極めて小さく、透過波の減衰はないと考える。運動学的回折は試料が非常に薄い場合(＜3 nm)にのみ適用できる。（b）動力学的回折では、入射波から回折が繰り返し起こり、試料がある厚さになると透過波の強度の全てが回折波に移ってしまう。そうすると、今度は回折波から透過波に向かって回折が起こる。ある厚さξg (消衰距離)に達すると、回折波の大きさは再びゼロになり、透過波の大きさは最大に戻る。このような透過波と回折波の強弱を繰り返す現象は、楔形の結晶で等厚干渉縞として観察される。

Electrons incident on a crystalline specimen are reflected (diffracted) by the lattice planes satisfying a Bragg condition. When the specimen is thick, many electrons are reflected. Then, the intensity of the incident wave decreases and that of the reflected wave exceeds that of the incident wave. At a certain thickness (half the extinction distance ξg), the incident wave intensity eventually becomes to zero, and the reflected wave reaches its maximum. Then, the reflected wave begins to be diffracted back toward the incident direction. When the thickness reaches the extinction distance ξg, the incident wave regains its maximum intensity, and the reflected wave intensity becomes back to zero. This interaction between the incident and diffracted waves is called dynamical diffraction.

When a specimen is very thin (<3 nm), diffraction phenomena can be treated under the assumption that only a minimal amount of reflection occurs without attenuation of the incident beam. This approximation is referred to as kinematical diffraction. It is noted that in X-ray and neutron diffraction the kinematical diffraction approximation can be applied due to their low scattering power, thereby complicated dynamical diffraction can be neglected.

The figure schematically illustrates kinematical diffraction, in which only minimal reflection occurs (Fig.(a)), and dynamical diffraction, in which many electrons are reflected and interactions arise between the incident and reflected waves (Fig. (b)).

Fig.(a) In kinematical diffraction, only minimal diffraction occurs from the incident wave, and the amplitude of the diffracted wave is very small compared to that of the transmitted wave, which is considered to experience no attenuation. Kinematical diffraction is applicable only when the specimen is very thin (<3 nm). (b) In dynamical diffraction, the incident wave undergoes repeated diffraction. When a certain depth is reached, the transmitted wave is entirely transferred to the diffracted wave. Then, diffraction occurs in the reverse direction from the diffracted wave back to the transmitted wave. When the depth reaches a depth ξg (extinction distance), the intensity of the diffracted wave returns to zero, and that of the transmitted wave regains to maximum. This oscillation between the transmitted and diffracted waves is observed as the equal thickness fringes in a wedge-shaped crystal.