タイコグラフィー

Ptychography

入射電子プローブを、その照射領域の一部が重なるよう試料上で二次元スキャンして各走査点において回折図形を取得し、回折図形の強度から試料の構造を再構成する方法。Ptychoはギリシャ語で重なり (fold) を意味する。X線構造解析の分野で実用的に使われている。
透過電子顕微鏡においては、装置の安定性向上と収差補正装置の開発とともに、回折図形の二次元デジタル画像を高速に取得できる高速高感度カメラの登場によって、より精度の高いデータ取得が可能となったことから、2012年頃から原子分解能の構造像を得る (位相回復) 手法の一つとして注目されてきた。特に近年の研究で、ノイズが少なくコントラストの高い構造像 (図2) が得られることが報告され、関心を集めている。透過電子顕微鏡では、以下の二つの方法のタイコグラフィーが現在行われている。

1) 入射電子線を試料上でデフォーカスして照射した状態で走査する方法 (図(a))
デフォーカスして照射領域を大きくした入射電子線を用いて、照射領域の一部が重なるように走査する。走査点数は、照射領域とプローブサイズに応じて数10点 ×数10点以下である。タイコグラフィーにより構造像 (位相回復像) を得る計算は以下のようにして行う。
試料の初期関数を1と仮定し、プローブ関数として箱型関数を仮定する。試料の出射波関数 (試料関数とプローブ関数の積) をフーリエ変換して回折図形を得る。この回折図形の強度を実験で取得した回折図形の強度と置き換える。更新された回折図形を逆フーリエ変換して実空間の像（構造像）に戻す。この操作によりこの試料位置での出射波関数が更新される。プローブ関数を正しい関数 (初期に与えた関数) に置き換え、この計算を繰り返す。その後、次のプローブ位置に移動して、同様の計算を実行する。このようなプロセスを順番に行い、計算による回折図形と実験で得た回折図形の差分が十分に小さくなるまで繰り返す。
このようにして得られた試料の構造像 (位相像) を図(c)に示す。本手法は従来のインコヒーレントなディフラクティブイメージングと類似しているが、隣り合う照射領域の重なり部分で試料関数が一致することが拘束条件に加わっているために、ディフラクティブイメージングで起きている解の一意性の問題は克服されている。さらに観察領域も走査することによって制限されなくなっている。

2) 入射電子線を試料上にフォーカスして照射した状態で走査する方法 (図(b))
試料上にフォーカスした電子線を用いて収束電子回折図形を二次元画像として取得する。その電子線を試料上で二次元的に走査する。通常、走査点は数万点を超え、取得したデータは四次元 (二次元走査＋二次元回折図形) の大量のデータとなる。
まず、[二次元走査R＋二次元回折図形K]の四次元データRKを、二次元走査に関する部分Rについてフーリエ変換して [二次元周波数Q＋二次元回折図形K]の四次元データQKを得る。ある空間周波数qに対する回折図形Kの中の "透過波と回折波との干渉領域 (K')" には、構造のフーリエ成分q-が強度として表れている (図(b)下部)。次に、得られる構造像（位相像）のS/N比を改善するために、干渉領域 (K') 外の強度の振幅をゼロにする。さらに、透過波に対して対称に位置する回折波と透過波との干渉領域(K'') の強度の符号 (位相)を反転させ、干渉領域 (K') の強度と同位相にする。この操作によって、積分時に通常はK'とK''で相殺されてしてしまう信号を足し合わせて強度を増強できる。引き続いて、QK中の周波数成分q毎に二次元回折図形 (図(b)下部) K' とK'' の強度を積分し、二次元空間周波数図形Q' をつくる。最後に、Q’ を逆フーリエ変換して、試料(実空間)の構造像(位相像)を得る(図(d))。

(校閲 Dr. Peng Wang、南京大学)

⇒図1
(a)電子線の照射領域の一部が重なるようにして走査するタイコグラフィー。照射領域は数nm～数10nm程度で、走査点数は通常、数10点 ×数10点以下である。
(b)電子プローブ(0.3nm程度以下)を試料上にフォーカスして走査するタイコグラフィー。走査点数は通常のSTEMと同様に数100点 × 数100点程度。回折図形の取得には、高速高感度カメラ(ピクセル型STEM検出器)が用いられている。
(c) (a)の手法により再構成した単層MoS₂の位相像(構造像)。（データ提供：Dr. Peng Wang 南京大学）
(d) (b)の手法により再構成した単層グラフェンの位相像(構造像)。

⇒図2
インフォーカスのタイコグラフィー再構成によって得られた構造像(位相像)、および同時に取得されたADF像との比較。試料：単層グラフェン、加速電：200kV
(a) 四次元データより再構成されたグラフェンの構造像。ここでは原子位置を明るく表示している。
(b) 四次元データと同時取得された通常のADF像。
両者を比較すると、再構成された構造像の方が、像のS/Nおよびコントラストがともに高くなっているのが分かる。

Ptychography is a method to reconstruct the crystal structure (image) of a specimen from the diffraction patterns obtained from each point (area) scanned over a specimen using a convergent probe so that a part of the illuminated area overlaps. “Ptycho” means “fold” in Greek. This method has been used in X-ray crystal structural analysis.
Ptychography for Electron Microscopy has attracted attention as one of the method to obtain the structural image (phase recovery) of atomic resolution since about 2012, owing to the advent of a high-speed and high-sensitivity camera that achieves fast acquisition of a two-dimensional (2D) digital image, together with improvement of microscope stability and advancement of the aberration corrector. In particular, it has been reported in recent electron ptychography studies that low-noise and high-contrast structure images are obtained, thus gaining increased attention. In transmission electron microscopy, the following two types of ptycography methods are being conducted.

1) Method to scan a specimen with a defocused convergent electron probe (Fig. (a))
A specimen is illuminated with a defocused convergent electron probe to broaden the illumination area. The probe is scanned on the specimen so that the adjacent illumination areas are partially overlapped to each other. The scan points of the probe are normally a few 10 points × a few 10 points or less depending on the scanning area and probe size. The procedure to obtain a structure image (phase image) by means of this type of ptychography is as follows:
The initial specimen function is assumed equal to 1 and the probe function is assumed to be a box function. The specimen exit-wave function (a product of the specimen and probe functions) is Fourier-transformed to obtain a diffraction pattern, the intensities of which are replaced by those of the pattern acquired experimentally. The updated diffraction pattern is then transformed back to an image in the real space by an inverse Fourier transform, which gives a new revised exit-wave function for this probe position. The probe function obtained is replaced with the correct function or the original one. And the above procedure is repeated. Then, the calculation moves to the next position. Aforementioned procedure is repeated until the difference of the calculated and experimental diffraction patterns becomes sufficiently small.
The resultant structural image (phase image) of the specimen is shown in Fig. (c). The present method is similar to conventional incoherent diffractive imaging. It should be however noted that non-unique solution problem arising in diffractive imaging is overcome due to the additional constraint, in which the specimen function in the overlapping region of the adjacent illumination areas have to be the same in the calculation for the diffraction patterns recorded. Furthermore, the issue of a limited field of view is cleared by scanning technique.

2) Method to scan a specimen with a focused convergent electron probe (Fig. (b))
A specimen is illuminated with a focused convergent electron probe．The convergent beam electron diffraction (CBED) pattern is recorded as a 2D image. The probe is scanned two dimensionally on the specimen. Scan points of the probe becomes a huge four-dimensional (4D) data set (2D CBED patterns +2D scan points), normally exceeding a few 10 thousands points.
The procedure for reconstructing a high-contrast structure image is as follows. First, the 4D data set RK (2D scan points R + 2D CBED patterns K) is Fourier-transformed with respect to the 2D scan points R to obtain another 4D data set QK (2D spatial frequencies Q + 2D CBED patterns K). In the overlapping area (K’) of the transmitted disc and a neighboring diffracted disc of the CBED pattern for a certain 2D spatial frequency q, the intensity of the Fourier component q of the structure appears (bottom of Fig. (b)). To improve the signal-to-noise ratio of the structure image (phase image) to be obtained, the intensities outside the interference area (K’) are set to be 0 (zero). And the sign (phase) of the intensities in the interference area (K'') located symmetrically with respect to the transmitted disc is reversed. By this processing, the intensities of the two areas (K’ and K''), which are normally canceled out when integrating, can be added positively or enhanced. Then, the intensities of the two areas (K’ and K'') of the 2D CBED pattern (bottom of Fig. (b)) are integrated for each spatial frequency component q of the 4D data set QK, so that a 2D spatial frequency pattern Q' is created. Finally, this 2D spatial frequency pattern Q' is inverse-Fourier transformed to obtain the structure image (phase image) of the specimen (Fig. (d)).

(Proofread by Dr. Peng Wang, Nanjing University)

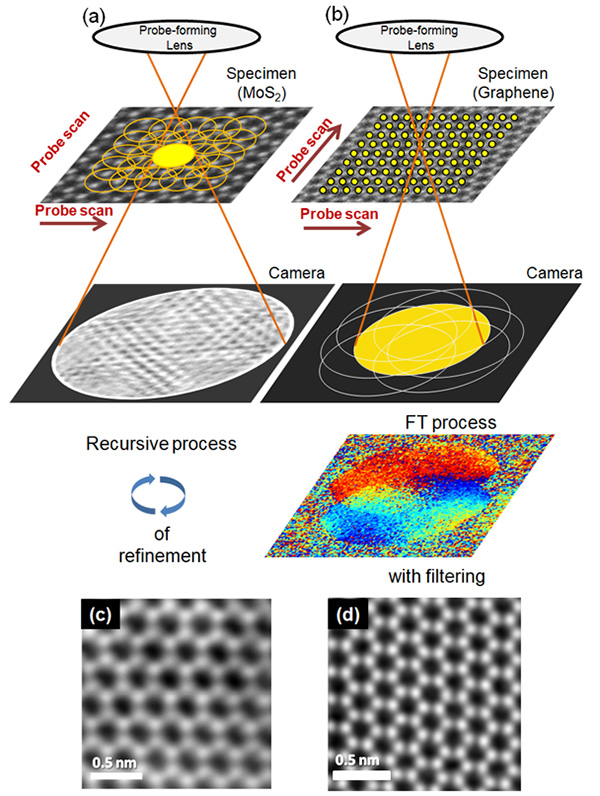
Fig. 1
(a) Ptychography in which a specimen is scanned with a defocused convergent electron probe so that adjacent illumination areas are partially overlapped to each other. The illumination area is about a few nm to a few 10 nm in diameter. The number of the scan points is normally a few 10 times a few 10 or less.
(b) Ptychography in which a specimen is scanned with a focused convergent electron probe (probe diameter: about 0.3 nm or less). The number of the scan points is a few 100 times a few 100 (the total number exceeding a few 10 thousands) like the case of ordinary STEM. A high-speed and high-sensitivity camera (pixelated STEM detector) is used to obtain a series of the CBED patterns.
(c) Structure image (Phase image) of a mono-layer of MoS₂ reconstructed by the defocus method (a). (Data courtesy: Dr. Peng Wang, Nanjing University)
(d) Structure image (Phase image) of a mono-layer graphene reconstructed by the focus method (b).

Fig. 2 Comparison of a reconstructed structure image (phase) obtained by Ptychography in which a specimen is scanned with a convergent probe and a simultaneously-obtained ABF image of a mono-layer graphene acquired at an accelerating voltage of 200 kV.
(a) Structure image (phase) of graphene reconstructed from 4D data set. Here, the atomic sites appear bright.
(b) Ordinal ADF image simultaneously acquired with the 4D data set.
Comparison of the two images elucidates that the reconstructed structure image (phase) has a higher signal-to-noise ratio and provides higher contrast than those of the ADF image.