ファイローゼット法（φ（ρz）法）

Phi-Rho-Z method (φ(ρz) method), PRZ

[目次：分析]

特性X線を用いた定量分析(試料を構成している元素の質量濃度を求めること）における、定量補正法の一つ。特性X線の発生量の深さ分布（発生関数φ(ρz)、ρは質量密度、ｚは深さ、ρzは質量深さという）を用いることで、ZAF補正では別々に扱われていた原子番号効果や吸収効果を同時に考慮することができ、精度の高い補正が可能になる。特に、重元素と共存している軽元素を分析する場合や、加速電圧が高く試料内のより深い部分から特性X線が発生する場合といった、吸収効果が大きい条件においてZAF補正より精度の高い定量分析ができる。

バルク試料を、厚さΔ(ρz)の薄膜が層状に重なったものとして考える。ある質量深さρzで発生する特性X線の強度ΔI(ρz)は(図 1の左図）、電子線が質量深さρzに到達するまでに散乱を受けるため、厚さΔ(ρz)の1枚の薄膜から発生する特性X線強度ΔI（図 1の右図）に質量深さρzでの発生関数φ（ρz）を掛ける必要がある。

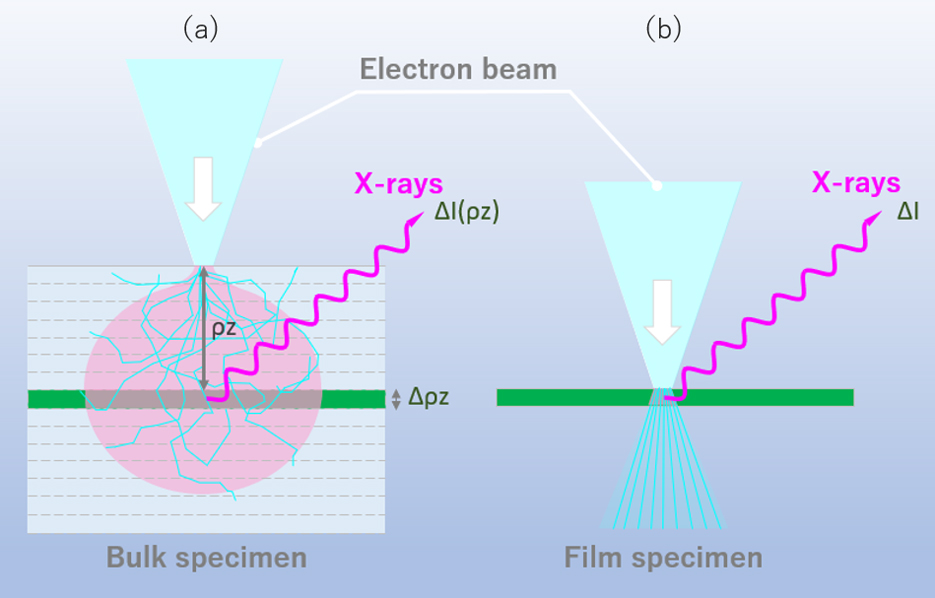
図 1 バルク試料と薄膜試料における照射電子線量の違い。

(a)バルク試料、(b)薄膜試料。バルク試料の場合、入射した電子線は質量深さρzに達するまでに散乱を受ける。そのため、薄膜と同じ厚さΔ(ρz)の層から発生する特性X線の強度ΔI(ρz)は、薄膜で発生する特性X線強度ΔIと異なり、発生関数を掛ける必要がある。

発生関数φ(ρz)のモデル式はいくつか提案されており、一般的には、ρzに対するφの変化は図 2のような形状をしている。ある質量深さ(ρz _m)まではφ(ρz)は増加する。その理由は、散乱により電子の方向が変えられるため、各層を通過する際の電子線の距離が長くなることにより特性X線を励起する確率が上がること、および後方散乱により深い層から戻ってきた電子も励起に寄与するためである。ρz _mより深い位置では、φ(ρz)は減少する。これは、散乱によりその深さまで侵入する電子の数が少ないことと、電子のエネルギーが減衰し特性X線を励起できなくなることに因る。

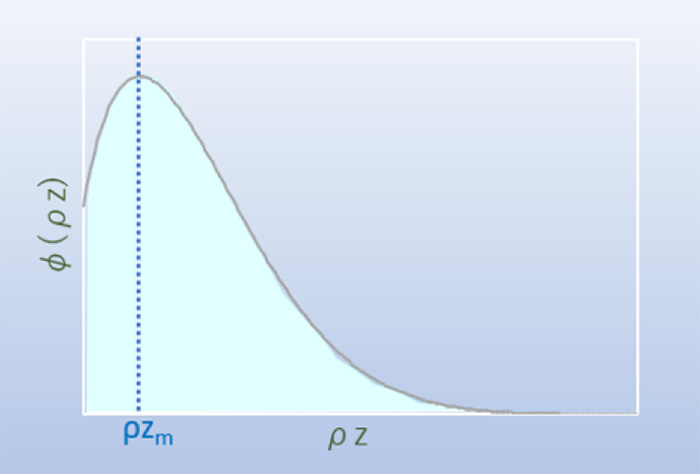
図 2　発生関数φ(ρz)

特性X線の発生関数φ(ρz)の質量深さρz依存性。一般的にはある質量深さρz _mまでは増加し、それ以降は減少する。

ある質量深さρzで発生する元素Aの特性X線強度ΔI_A(ρz)は、質量濃度C_Aを考慮すると、

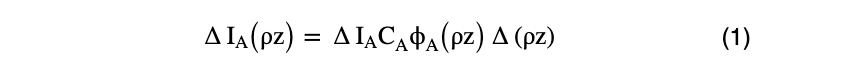
とあらわされる。ただし、ΔI_Aは質量厚さΔ(ρz)の一枚の薄膜試料から放出される元素Aの特性X線強度、C_Aは元素Aの質量濃度[mass%]、φ_A(ρz)は元素Aに対する発生関数、ρは試料の質量密度、zは試料の深さである。

さらに、発生した特性X線 ΔI_A(ρz)は、試料外に放出されるまでに、試料内を通過する距離に応じた吸収を受ける(図 3)。吸収の大きさは、試料の質量吸収係数や検出器の取り出し角を考慮したX線の通過距離に依存し、減衰する指数関数で表される。その結果、試料外の検出器に向かう特性X線の強度ΔI’_A(ρz)は、

となる。ただし、[μ/ρ]は元素Aの特性X線に対する試料内での質量吸収係数、θは試料表面に対する検出器の取出角度である。

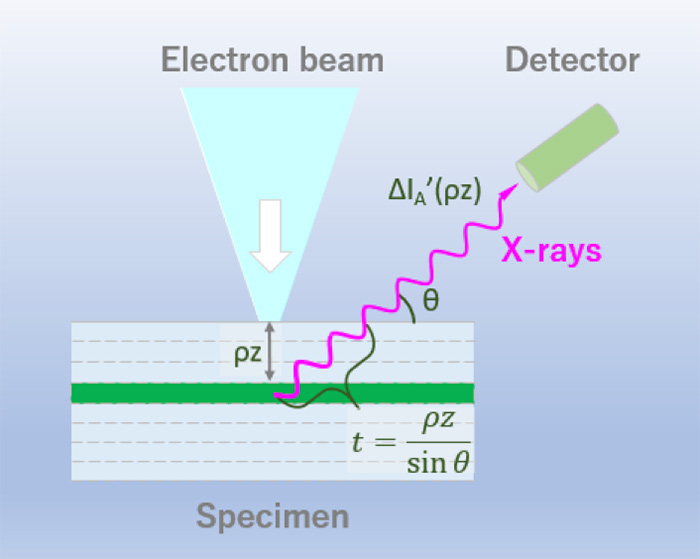
図 3　特性X線が検出されるまでに試料内を通過する距離

ある質量深さρzで発生した特性X線は、検出器に入射するまでに試料内の t = ρz/sinθ の距離を通過する。

検出される特性X線強度I’_Aは、式(2)を質量深さρzについて積分することにより求めることができ、

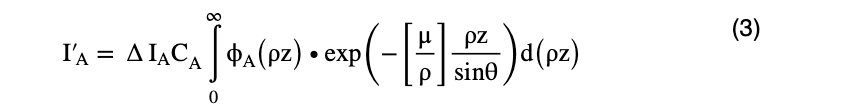
となる。
よって、標準試料(化学組成が既知の試料)と未知試料の、検出される特性X線強度の比は、以下のように表される。

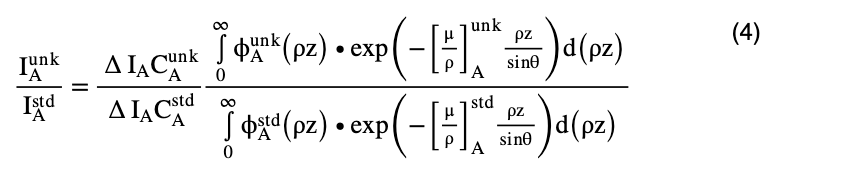
ただし、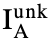は未知試料から検出される元素Aの特性X線強度、は標準試料から検出される元素Aの特性X線強度、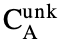は未知試料中の元素Aの質量濃度、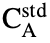は標準試料中の元素Aの質量濃度、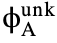(ρz)は未知試料の元素Aの発生関数、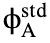(ρz)は標準試料の元素Aの発生関数、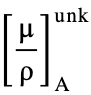は未知試料における元素Aの特性X線に対する質量吸収係数、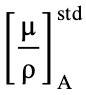は標準試料における元素Aの特性X線に対する質量吸収係数を示す。

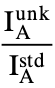 = K(Kレシオ)なので、以下のように変形できる。

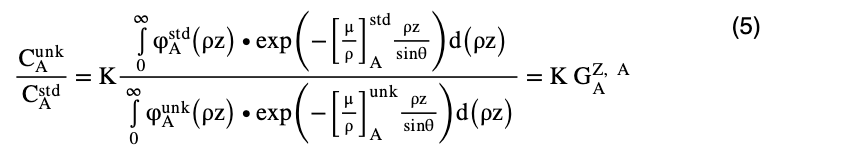
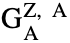が、φ(ρz)法における、原子番号効果および吸収効果の補正項である。
さらに、ZAF補正と同様に、蛍光補正(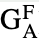)を考慮することによって、φ(ρz)法の定量補正係数(G)は

となる。

The Phi-Rho-Z method (φ(ρz) method) is one of quantitative correction methods in obtaining the mass concentrations of the constituent elements of a specimen using characteristic X-rays generated by an electron beam.

The method uses the depth distribution of the generated characteristic X-rays, where the atomic number (Z) effect and the absorption (A) effect are simultaneously taken into account. Then, the φ(ρz) method provides a higher accuracy quantitative correction than the ZAF correction method in which the both effects are separately treated. In particular, the φ(ρz) method can provide more accurate results than ZAF correction especially in the cases requiring accurate absorption correction; for the specimens consisting of light elements and heavy elements, and for the high accelerating voltages of the incident electron beam where the characteristic X-rays are generated over a deep region in the specimen. The depth distribution of the characteristic X-rays is given by a functionφ(ρz), where ρ is the mass density, z is the depth from the specimen surface and then ρz expresses the mass depth.

Consider a bulk specimen to be composed of stacking of thin layers with each thickness Δ(ρz). The intensity of the characteristic X-rays ΔI(ρz) generated from a layer at a certain mass depth ρz (left figure in Fig. 1) is different from the intensity of the characteristic X-rays ΔI generated from a single thin film with the same mass thickness (right figure in Fig. 1), and needs to multiply the generation function φ(ρz) to the intensity ΔI because the electron beam is scattered before reaching the layer at the mass depth ρz.

Fig. 1 Difference of the intensities of the generated characteristic X-rays between a bulk specimen (a) and a single thin-film specimen (b). In a bulk specimen, the incident electron beam is scattered until the beam reaches the layer at the mass depth ρz. Thus, the intensity of the characteristic X-rays ΔI(ρz) generated at depth Δ(ρz) needs to multiply the generation function φ(ρz) to the intensity of the characteristic X-rays ΔI from a single thin-film specimen.

Some model functions for φ(ρz) have been proposed. They have such ρz dependence as shown in Fig. 2. That is, the value of φ(ρz) increases up to the mass depth (ρz _m) due to the fact that an electron beam changes their travelling direction by scattering and the flight distance in each layer becomes longer to increase the exciting probability of the characteristic X-rays. Also, backscattering from a deeper region of the specimen contributes to increase the X-ray generation. The value ofφ (ρz) decreases when the depth is greater than ρz _m because, by scattering, the number of the penetrating electrons decreases and the penetrating electron energies become small to excite the characteristic X-rays.

Fig. 2 Generation function φ(ρz)

Dependence of the characteristic X-ray generation function φ(ρz) on the mass depth (ρz). The function increases up to a certain mass depth ρz _m, and decreases beyond the depth.

The intensity of the characteristic X-raysΔI_A(ρz) from element A generated at a certain mass depth ρz is expressed by the following equation,

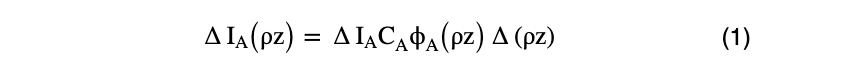
Here, ΔI_A is the intensity of the characteristic X-rays of element A emitted from the thin-film specimen with mass thickness Δ(ρz), C_A is the mass concentration [mass%] of element A, φ_A(ρz) is the generation function for element A, ρ is the mass density of the specimen, and z is the depth of the specimen.

The intensity of the characteristic X-rays ΔI_A(ρz) suffers absorption which depends on the path length of the characteristic X-rays in the specimen until those X-rays are emitted out of the specimen (Fig. 3). The magnitude of absorption depends on the mass absorption coefficient of the specimen and on the path length of the X-rays travelling toward the X-ray detector, and is expressed by an attenuated exponential function. Thus, the intensity of the characteristic X-rays ΔI’_A(ρz) measured by the X-ray detector (placed outside the specimen) is expressed as

Here, [μ/ρ] is the mass absorption coefficient for the characteristic X-rays of element A in the specimen, and θ is the take-off angle of the X-ray detector against the specimen surface.

Fig. 3 Path length of the characteristic X-rays in the specimen toward the X-ray detector. The path length is expressed as t = ρz/sinθ, where ρz is the mass depth and θ is the take-off angle of the X-ray detector against the specimen surface.

The intensity of the characteristic X-rays ΔI’_A detected is obtained by integrating the equation (2) with respect to the mass depth ρz, and expressed as

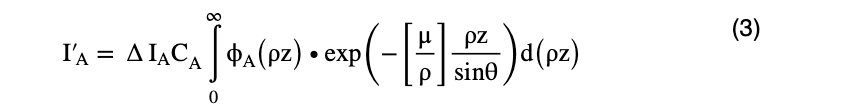
Thus, the detected characteristic X-ray intensity ratio of the unknown specimen to that of the standard specimen (chemical composition is known) is expressed by the following equation.

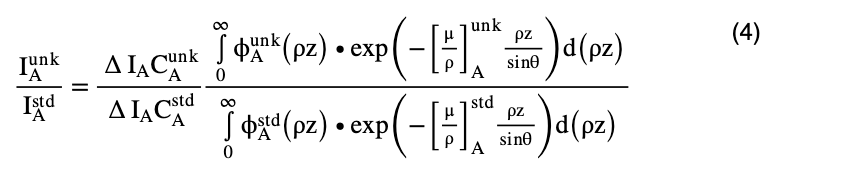
Here, 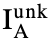is the characteristic X-ray intensity of element A detected from the unknown specimen, and is the characteristic X-ray intensity of element A detected from the standard specimen. 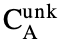is the mass concentration of element A in the unknown specimen, and 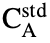is the mass concentration of element A in the standard specimen. 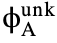(ρz) is the generation function of element A in the unknown specimen, and 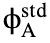(ρz) is the generation function of element A in the standard specimen. 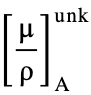is the mass absorption coefficient for the characteristic X-ray of element A in the unknown specimen, and 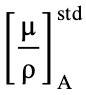is the mass absorption coefficient for the characteristic X-ray of element A in the standard specimen.

Then,
Using the equation 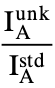 = K (K ratio), the equation (4) is rewritten as

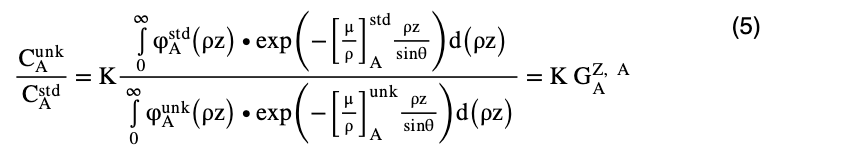
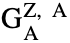is the correction factor for the atomic number effect and the absorption effect in the φ(ρz) method. In addition, like the case of ZAF correction, by taking into account the fluorescence correction factor (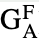), the quantitative correction factor (G) in the φ(ρz) method finally becomes

関連用語から探す